Introduzione
Abstract
En
Given a countably paracompact normal space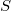 with a closed subspace
with a closed subspace 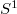 and a finite derected graph
and a finite derected graph  with a subgraph
with a subgraph 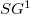 , there are bijections from the sets of homotopy classes
, there are bijections from the sets of homotopy classes  and
and 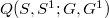 to the sets of o*-homotopy classes
to the sets of o*-homotopy classes 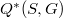 and
and 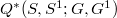 respectively (see 3).Here we prove,by some examples,that certain conditions on
respectively (see 3).Here we prove,by some examples,that certain conditions on 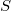 weaker than the ones required in (3) are not sufficent to prove the results given above.
weaker than the ones required in (3) are not sufficent to prove the results given above.
Given a countably paracompact normal space
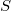 with a closed subspace
with a closed subspace 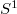 and a finite derected graph
and a finite derected graph  with a subgraph
with a subgraph 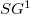 , there are bijections from the sets of homotopy classes
, there are bijections from the sets of homotopy classes  and
and 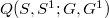 to the sets of o*-homotopy classes
to the sets of o*-homotopy classes 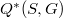 and
and 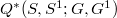 respectively (see 3).Here we prove,by some examples,that certain conditions on
respectively (see 3).Here we prove,by some examples,that certain conditions on 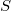 weaker than the ones required in (3) are not sufficent to prove the results given above.
weaker than the ones required in (3) are not sufficent to prove the results given above.DOI Code:
§
Full Text: PDF


