Generalized Fourier expansions for zero-solutions of surjective convolution operators on  and
and 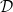
Abstract
It is well-known that each distribution 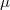 with compact support can be convolved with an arbitrary distribution and that this defines a convolution operator
with compact support can be convolved with an arbitrary distribution and that this defines a convolution operator 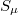 , acting on
, acting on  .The surjectivity of
.The surjectivity of 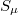 , was characterized by Ehrenpreis [5]. Extending this result,we characterize in the present article the surjectivity of convolution operators on the space
, was characterized by Ehrenpreis [5]. Extending this result,we characterize in the present article the surjectivity of convolution operators on the space  of all w-ultradistributions of Beurling type on R.This is done in two steps. In the first one we show that
of all w-ultradistributions of Beurling type on R.This is done in two steps. In the first one we show that 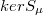 has an absolute basis whenever
has an absolute basis whenever 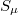 , admits a fundamental solution
, admits a fundamental solution 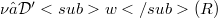 . The expansion of an element in
. The expansion of an element in 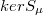 , with respect to this basis can be regarded as a generalization of the Fourier expansion of periodic ultradistributions.In the second step we use this sequence space representation together with results of Palamodov [15] and Vogt [17], [18] on the projective limit functor to obtain the desired characterization.It turns out that
, with respect to this basis can be regarded as a generalization of the Fourier expansion of periodic ultradistributions.In the second step we use this sequence space representation together with results of Palamodov [15] and Vogt [17], [18] on the projective limit functor to obtain the desired characterization.It turns out that 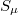 is surjective if and only if
is surjective if and only if 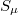 admits a fundamental solution. Hence the elements of
admits a fundamental solution. Hence the elements of 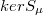 admit a generalized Fourier expansion for each surjective convolution operator
admit a generalized Fourier expansion for each surjective convolution operator 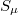 on
on  .Note that this differs from the behavior of convolution operators on the space
.Note that this differs from the behavior of convolution operators on the space  of w-ultradifferentiable functions of Roumieu-type, as Braun,Meise and Vogt [4] have shown. Note also that the results of the present article apply to convolution operators on
of w-ultradifferentiable functions of Roumieu-type, as Braun,Meise and Vogt [4] have shown. Note also that the results of the present article apply to convolution operators on  ,too.
,too.
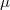 with compact support can be convolved with an arbitrary distribution and that this defines a convolution operator
with compact support can be convolved with an arbitrary distribution and that this defines a convolution operator 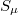 , acting on
, acting on  .The surjectivity of
.The surjectivity of 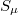 , was characterized by Ehrenpreis [5]. Extending this result,we characterize in the present article the surjectivity of convolution operators on the space
, was characterized by Ehrenpreis [5]. Extending this result,we characterize in the present article the surjectivity of convolution operators on the space  of all w-ultradistributions of Beurling type on R.This is done in two steps. In the first one we show that
of all w-ultradistributions of Beurling type on R.This is done in two steps. In the first one we show that 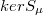 has an absolute basis whenever
has an absolute basis whenever 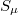 , admits a fundamental solution
, admits a fundamental solution 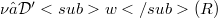 . The expansion of an element in
. The expansion of an element in 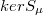 , with respect to this basis can be regarded as a generalization of the Fourier expansion of periodic ultradistributions.In the second step we use this sequence space representation together with results of Palamodov [15] and Vogt [17], [18] on the projective limit functor to obtain the desired characterization.It turns out that
, with respect to this basis can be regarded as a generalization of the Fourier expansion of periodic ultradistributions.In the second step we use this sequence space representation together with results of Palamodov [15] and Vogt [17], [18] on the projective limit functor to obtain the desired characterization.It turns out that 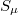 is surjective if and only if
is surjective if and only if 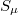 admits a fundamental solution. Hence the elements of
admits a fundamental solution. Hence the elements of 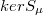 admit a generalized Fourier expansion for each surjective convolution operator
admit a generalized Fourier expansion for each surjective convolution operator 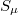 on
on  .Note that this differs from the behavior of convolution operators on the space
.Note that this differs from the behavior of convolution operators on the space  of w-ultradifferentiable functions of Roumieu-type, as Braun,Meise and Vogt [4] have shown. Note also that the results of the present article apply to convolution operators on
of w-ultradifferentiable functions of Roumieu-type, as Braun,Meise and Vogt [4] have shown. Note also that the results of the present article apply to convolution operators on  ,too.
,too.DOI Code:
10.1285/i15900932v10supn1p251
Full Text: PDF


