(DF)-Spaces of type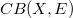 
and 
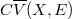
Abstract
Some locally convex properties of the spaces 
of the bounded continuous functions on a completely regular Hausdorff space X with values in a (DF-space) E are studied and applied to the (DF)-spaces of type 
(e.g., see [S]).The following are our main results:
1.
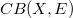is a (DF)-space if and only if E is a (DF)-space.
2.For a (DF)-space E, 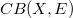is quasi barrelled if and only if either (i)X is pseudocompact and E is quasibarrelled or (ii) X is not pseudocompact and the bounded subsets of E are metrizaable.
3. If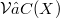 
and if each 
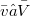is dominated by some 
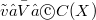, then (resp.,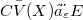 
) is a (DF)-space if and only if E is a (DF)-space.
4. Let X be a locally compact and σ-compact space, 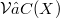and E a (DF)-space. Then is quasibarrelled if and only if (i) E is quasibarrelled and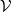 
satisfies condition 
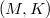or (ii) the bounded subsets of E are metrizable and 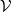satisfies condition (D).
of the bounded continuous functions on a completely regular Hausdorff space X with values in a (DF-space) E are studied and applied to the (DF)-spaces of type (e.g., see [S]).The following are our main results:
1.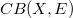 is a (DF)-space if and only if E is a (DF)-space.
2.For a (DF)-space E, 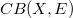is quasi barrelled if and only if either (i)X is pseudocompact and E is quasibarrelled or (ii) X is not pseudocompact and the bounded subsets of E are metrizaable.
3. If 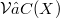and if each 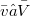is dominated by some 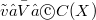, then (resp., 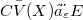) is a (DF)-space if and only if E is a (DF)-space.
4. Let X be a locally compact and σ-compact space, 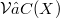and E a (DF)-space. Then is quasibarrelled if and only if (i) E is quasibarrelled and 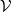satisfies condition 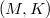or (ii) the bounded subsets of E are metrizable and satisfies condition (D).DOI Code:
10.1285/i15900932v10supn1p127
Full Text: PDF
