Quotients of Raikov-complete topological groups
Abstract
A topological group X is called Raikov-complete if the two sides uniformity on X, that is the supremum 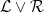 of the left uniformity and the right uniformity
of the left uniformity and the right uniformity 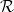 on X, is complete.It will be proved that the quotient
on X, is complete.It will be proved that the quotient 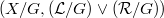 of an inframetrizable Raikov-complete topological group X with a neutral subgroup G is complete.(If G is normal (and therefore neutral), the completeness of
of an inframetrizable Raikov-complete topological group X with a neutral subgroup G is complete.(If G is normal (and therefore neutral), the completeness of 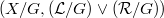 is equivalent to the Raikov-completeness of the quotient group
is equivalent to the Raikov-completeness of the quotient group  ).The proof consist in an intricate lifting of
).The proof consist in an intricate lifting of 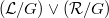 -Cauchy filters. Where it is possible, the results on topological groups will be derived from results on uniform spaces.
-Cauchy filters. Where it is possible, the results on topological groups will be derived from results on uniform spaces.
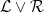 of the left uniformity and the right uniformity
of the left uniformity and the right uniformity 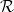 on X, is complete.It will be proved that the quotient
on X, is complete.It will be proved that the quotient 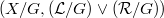 of an inframetrizable Raikov-complete topological group X with a neutral subgroup G is complete.(If G is normal (and therefore neutral), the completeness of
of an inframetrizable Raikov-complete topological group X with a neutral subgroup G is complete.(If G is normal (and therefore neutral), the completeness of 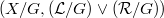 is equivalent to the Raikov-completeness of the quotient group
is equivalent to the Raikov-completeness of the quotient group  ).The proof consist in an intricate lifting of
).The proof consist in an intricate lifting of 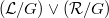 -Cauchy filters. Where it is possible, the results on topological groups will be derived from results on uniform spaces.
-Cauchy filters. Where it is possible, the results on topological groups will be derived from results on uniform spaces.DOI Code:
10.1285/i15900932v13n1p75
Classification:
54E15; 54E50; 22A05
Full Text: PDF


