Heegaard splittings of the Brieskorn homology spheres that are equivalent after one stabilization
Abstract
We construct a class of 3-manifolds 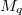 which are homeomorphic to the Brieskorn homology spheres
which are homeomorphic to the Brieskorn homology spheres 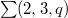 , where
, where 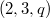 are relatively prime. Also, we show that
are relatively prime. Also, we show that 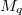 is a
is a 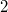 -fold cyclic branched covering of
-fold cyclic branched covering of 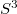 over a knot
over a knot 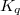 which is inequivalent with torus knot
which is inequivalent with torus knot 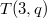 for
for 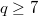 . Moreover, we show that two inequivalent Heegaard splittings of
. Moreover, we show that two inequivalent Heegaard splittings of 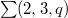 of genus 2 associated with
of genus 2 associated with 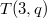 and
and 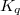 are equivalent after single stabilization.
are equivalent after single stabilization.
DOI Code:
10.1285/i15900932v20n1p53
Keywords:
Crystallization; Heegaard splitting; Crystallization move; Brieskorn homology sphere
Classification:
57M12; 57M15; 57M50
Full Text: PDF


