Selection principles and the Minimal Tower problem
Abstract
We study diagonalizations of covers using various selectionprinciples, where the covers are related to linearquasiorderings ( -covers).This includes: equivalences and nonequivalences,combinatorial characterizations, critical cardinalities andconstructions of special sets of reals.This study leads to a solution of a topological problem which wassuggested to the author by Scheepers (and stated in [15]) and is related to the Minimal Tower problem.
-covers).This includes: equivalences and nonequivalences,combinatorial characterizations, critical cardinalities andconstructions of special sets of reals.This study leads to a solution of a topological problem which wassuggested to the author by Scheepers (and stated in [15]) and is related to the Minimal Tower problem.
We also introduce a variant of the notion of  -cover,called
-cover,called 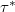 -cover, and settle some problems for thisvariant which are still open in the case of
-cover, and settle some problems for thisvariant which are still open in the case of  -covers.This new variant introduces new (and tighter) topologicaland combinatorial lower bounds on the Minimal Tower problem.
-covers.This new variant introduces new (and tighter) topologicaland combinatorial lower bounds on the Minimal Tower problem.
DOI Code:
10.1285/i15900932v22n2p53
Keywords:
Gerlits-Nagy property $gamma$-sets; $gamma$-cover; $omega$-cover; $ au$-cover; Tower; Selection principles; Borel covers; Open covers
Classification:
03E05; 54D20; 54D80
Full Text: PDF


