The Pytkeev property and the Reznichenko property in function spaces
Abstract
For a Tychonoff space  we denote by
we denote by 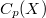 the space of all real-valued continuous functions on
the space of all real-valued continuous functions on  with the topology of pointwise convergence. Characterizations of sequentiality and countable tightness of
with the topology of pointwise convergence. Characterizations of sequentiality and countable tightness of 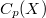 in terms of
in terms of  were given by Gerlits, Nagy, Pytkeev and Arhangel'skii. In this paper, we characterize the Pytkeev property and the Reznichenko property of
were given by Gerlits, Nagy, Pytkeev and Arhangel'skii. In this paper, we characterize the Pytkeev property and the Reznichenko property of 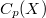 in terms of
in terms of  . In particular we note that if
. In particular we note that if 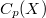 over a subset
over a subset  of the real line is a Pytkeev space, then
of the real line is a Pytkeev space, then  is perfectly meager and has universal measure zero.
is perfectly meager and has universal measure zero.
DOI Code:
10.1285/i15900932v22n2p43
Keywords:
Function space; Topology of pointwise convergence; Sequential; Countable tightness; Pytkeev space; Weakly Fréchet-Urysohn; $omega$ -cover; $omega$-shrinkable; $omega$-grouping property; The Menger property; The Rothberger property; The Hurewicz property; Universal measure zero; Perfectly meager; Property $(gamma)$
Classification:
54C35; 54D20; 54D55; 54H05
Full Text: PDF


