Total subspaces with long chains of nowhere norming weak*-sequential closures
Abstract
If a separable Banach space X is such that for some nonquasireflexive Banach space Y there exists a surjective stricty singular operator  then for every countable ordinal
then for every countable ordinal 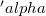 the dual of X contains a subspace whose weak* sequential closures of orders less than
the dual of X contains a subspace whose weak* sequential closures of orders less than 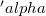 are nowhere norming and whose weak* sequential closure of order
are nowhere norming and whose weak* sequential closure of order 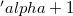 coincides with
coincides with  .
.
 then for every countable ordinal
then for every countable ordinal 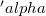 the dual of X contains a subspace whose weak* sequential closures of orders less than
the dual of X contains a subspace whose weak* sequential closures of orders less than 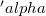 are nowhere norming and whose weak* sequential closure of order
are nowhere norming and whose weak* sequential closure of order 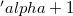 coincides with
coincides with  .
.DOI Code:
10.1285/i15900932v13n2p217
Full Text: PDF


