Higher order valued reduction theorems for general linear connections
Abstract
The reduction theorems for general linear and classical connections are generalized for operators with values in higher order gauge-natural bundles. We prove that natural operators depending on the 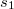 -jets of classical connections, on the
-jets of classical connections, on the 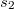 -jets of general linear connections and on the
-jets of general linear connections and on the  -jets of tensor fields with values in gauge-natural bundles of order
-jets of tensor fields with values in gauge-natural bundles of order  ,
, 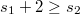 ,
, 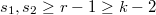 , can be factorized through the
, can be factorized through the 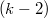 -jets of both connections, the
-jets of both connections, the 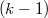 -jets of the tensor fields and sufficiently high covariant differentials of the curvature tensors and the tensor fields.
-jets of the tensor fields and sufficiently high covariant differentials of the curvature tensors and the tensor fields.
DOI Code:
10.1285/i15900932v23n2p75
Keywords:
Gauge-natural bundle; natural operator; Linear connection; Classical connection; Reduction theorem
Classification:
53C05; 58A20
Full Text: PDF


