Catene di cerchi ottenibili mediante punti pseudoregolari rispetto ad una conica di un piano di Galois
Abstract
Let Q be an elliptic quadric of 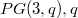 odd: the study of certain sets of
odd: the study of certain sets of 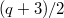 circles on Q, so-called chains, is important for the theory of translation planes (cfr.[1]). Here one studies the chains with the property that the planes of
circles on Q, so-called chains, is important for the theory of translation planes (cfr.[1]). Here one studies the chains with the property that the planes of 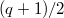 or
or  circles of the chains all meet in one point and one gaves various examples.
circles of the chains all meet in one point and one gaves various examples.
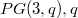 odd: the study of certain sets of
odd: the study of certain sets of 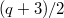 circles on Q, so-called chains, is important for the theory of translation planes (cfr.[1]). Here one studies the chains with the property that the planes of
circles on Q, so-called chains, is important for the theory of translation planes (cfr.[1]). Here one studies the chains with the property that the planes of 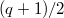 or
or  circles of the chains all meet in one point and one gaves various examples.
circles of the chains all meet in one point and one gaves various examples.DOI Code:
10.1285/i15900932v1n1p113
Full Text: PDF


