Of finite geometries of type 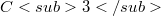 with thick lines
with thick lines
Abstract
Several facts suggest the conjecture that every finite geometry of type 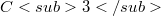 with thick lines is either a bulding or flat ( see [4] , [5] , [8] and [9]). That conjecture plays a central role in the problem of classifying finite thick geometries of type
with thick lines is either a bulding or flat ( see [4] , [5] , [8] and [9]). That conjecture plays a central role in the problem of classifying finite thick geometries of type 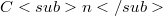 and
and 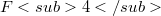 ( see [4] , [7] and [8] ).In this paper we find very simple conditions that hold in a finite geometry of type
( see [4] , [7] and [8] ).In this paper we find very simple conditions that hold in a finite geometry of type 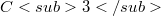 with thick lines if and only if the geometry is either a building or flat. Then the previous conjecture can be restated so: prove that the conditions discussed in this paper are theorems in the case of finite geometries with thick lines.
with thick lines if and only if the geometry is either a building or flat. Then the previous conjecture can be restated so: prove that the conditions discussed in this paper are theorems in the case of finite geometries with thick lines.
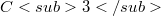 with thick lines is either a bulding or flat ( see [4] , [5] , [8] and [9]). That conjecture plays a central role in the problem of classifying finite thick geometries of type
with thick lines is either a bulding or flat ( see [4] , [5] , [8] and [9]). That conjecture plays a central role in the problem of classifying finite thick geometries of type 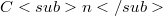 and
and 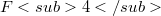 ( see [4] , [7] and [8] ).In this paper we find very simple conditions that hold in a finite geometry of type
( see [4] , [7] and [8] ).In this paper we find very simple conditions that hold in a finite geometry of type 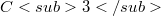 with thick lines if and only if the geometry is either a building or flat. Then the previous conjecture can be restated so: prove that the conditions discussed in this paper are theorems in the case of finite geometries with thick lines.
with thick lines if and only if the geometry is either a building or flat. Then the previous conjecture can be restated so: prove that the conditions discussed in this paper are theorems in the case of finite geometries with thick lines.DOI Code:
10.1285/i15900932v6n2p205
Full Text: PDF


