Spread-Theoretic Dual of a Semifield
Abstract
Given a finite pre-semifield 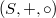 , the dual pre-semifield
, the dual pre-semifield  has multiplication
has multiplication 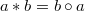 . In this article, acharacterization is given of the dual pre-semifield in terms of theassociated spreads. This is then used to give a new proof that shows thatself-dual pre-semifield spreads when transposed and dualized constructself-transpose semifield spreads. When the self-dual pre-semifield isactually commutative then the transpose-dual spread is symplectic. We alsogive a spread-only description of the six semifields arising from a givensemifield.
. In this article, acharacterization is given of the dual pre-semifield in terms of theassociated spreads. This is then used to give a new proof that shows thatself-dual pre-semifield spreads when transposed and dualized constructself-transpose semifield spreads. When the self-dual pre-semifield isactually commutative then the transpose-dual spread is symplectic. We alsogive a spread-only description of the six semifields arising from a givensemifield.
DOI Code:
10.1285/i15900932v29n1p145
Keywords:
51E23; 51A40
51E23; 51A40
Full Text: PDF


