On the Gauss map of embedded minimal tubes
Abstract
The Gaussian image of the minimal tubes of arbitrary dimension is studied. If the angle between the flow-vector of such a surface M and its axe is equal to 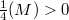 then the diameter of the Gauss image of M is at least
then the diameter of the Gauss image of M is at least 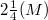 . As a consequence we show that the length of a two dimensional minimal tube M can be estimated by the angle
. As a consequence we show that the length of a two dimensional minimal tube M can be estimated by the angle  and the total Gaussian curvature of M.
and the total Gaussian curvature of M.
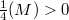 then the diameter of the Gauss image of M is at least
then the diameter of the Gauss image of M is at least 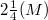 . As a consequence we show that the length of a two dimensional minimal tube M can be estimated by the angle
. As a consequence we show that the length of a two dimensional minimal tube M can be estimated by the angle  and the total Gaussian curvature of M.
and the total Gaussian curvature of M.DOI Code:
10.1285/i15900932v19n1p7
Keywords:
Minimal tubes; Gaussian map; Total gaussian curvature; Flow vector
Classification:
53A10; 53A55
Full Text: PDF


